.
I am interested mainly in applications of geometric ideas in Science. My main research topics lie within Einstein’s theory of gravity, the prototypical example of a geometric theory in fundamental Physics.
Conformal infinity

The frame-work for all the numerical efforts to compute wave forms for gravitational radiation is based on the notion of an isolated system. This is an idealisation of a real world situation such as a binary pulsar somewhere out there in the universe. The gravitating system consists of several individual components, which interact with each other thereby changing the fabric of space-time by changing its curvature. In contrast to Newton’s theory Einstein’s theory of gravity has no action at a distance which means that changes to the curvature are not transported instantaneously but travel with a finite speed. The task of gravitational wave detectors such as GEO600, LIGO and VIRGO/EGO is to pick up these changes in the space-time curvature when they pass by.
Integrable systems and Riemann surfaces
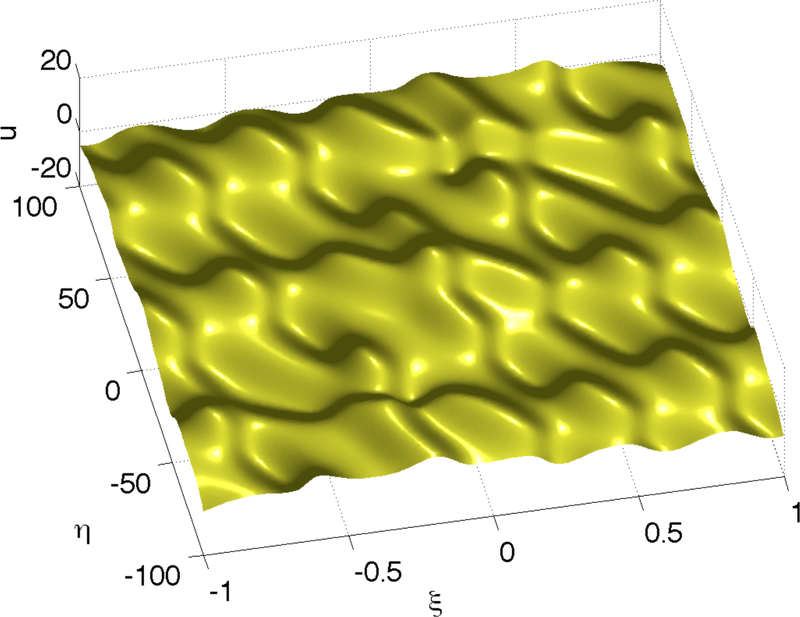
Riemann surfaces are one-dimensional complex manifolds. Their origin is in complex analysis where they serve as the natural domains of definition for multivalued analytic functions such as square roots. They can be constructed by using the principle of analytic continuation. From another point of view they are defined as the level set of a complex polynomial in two variables x and y. A remarkable body of work relates the structure of Riemann surfaces with integrable systems of partial differential equations. Famous examples of such equations are the Korteweg-deVries equation, the Kadomtsev-Petviashvili equation, the Davey-Stewartson equation and the Kamassa-Holm equation. These equations are all obtained from the equations of fluid dynamics under certain simplifications. More generally, it is conjectured that all integrable systems are symmetry reductions of the self-dual Yang-Mills equations. It turns out that solutions of these systems can be expressed in terms of theta-functions, which are defined on Riemann surfaces.
Air-Water interfaces (bubbles)

The picture on the left shows the situation that we wanted to understand: some plants have very small `hairs’ on the leaves which allow them to keep pockets of air attached to them even when they are submerged under water. The interface between the air pockets and the surrounding water is mathematically described as a surface of constant mean curvature. The appropriate equation for this situation is the Young-Laplace equation, which essentially describes the fact that the force acting upon an element of the interface due to surface tension must be compensated for by the difference of pressure from both sides of the interface. We wanted to model the leaf with hairs and the interface attached, which led us to a boundary-value problem for the Young-Laplace equation. The boundary conditions come from the structure of the hairs which have certain hydrophilic properties. They determine the contact angle between the hairs and the interface, i.e., mathematically speaking, they provide a Neumann condition for the problem. In the usual treatments one assumes that the interface can be described in terms of a height-function which gives the height of the surface above a given plane. However, when modeling the leaf with hairs this formulation is inappropriate and one needs to use a more general formulation. We chose the embedding formalism, where we assume the interface to be described as a surface patch for a surface embedded in R3.